Loi normale centrée réduite
Définition :
Dire qu'une variable aléatoire continue \(X\) suit la loi normale centrée réduite signifie que sa densité de probabilité est la fonction de Gauss f définie sur \(\mathbb R\) par :
\(f(x)=\dfrac{1}{\sqrt{2\pi}}e^{-\dfrac{x^2}{2}}\)
On note \(X\hookrightarrow \mathcal N(0 ;1)\).
Fondamental : Propriétés
\(\mathbb P(a\leqslant X \leqslant b)=\displaystyle \int_a^b ~\dfrac{1}{\sqrt{2\pi}}e^{-\dfrac{x^2}{2}}~dx\)
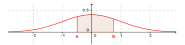
De plus : \(\mathbb P(a\leqslant X \leqslant b)=\mathbb P(a< X \leqslant b)=\mathbb P(a\leqslant X < b)=\mathbb P(a<X <b)\)
\(\mathbb E(X)=0\) et \(\sigma(X)=1\) d'où la dénomination centrée et réduite...
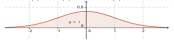
L'aire totale sous la courbe est égale à 1. Elle représente la probabilité \(\mathbb P(-\infty <X < +\infty)\) de l'événement certain.
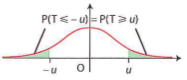
La courbe est symétrique par rapport à l'axe des ordonnées, donc :
\(\mathbb P(X\geqslant 0)=\dfrac{1}{2}\)
\(\mathbb P(X\geqslant u)=\mathbb P(X\leqslant -u)\) pour tout réel \(u\in \mathbb R\)